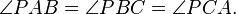~F N David: Games, God and Gambling (1962)
The 132nd day of the year; 132 and its reversal (231) are both divisible by the prime 11 (132/11 = 12, 231/11 = 21). Note that the resulting quotients are also reversals. *Prime Curios
If you take the sum of all 2-digit numbers you can make from 132, you get 132: These are called Osiros numbers, and there are only three using two digits of a three digit number. One is a year date, and one is a little too big.
12 + 13 + 21 + 23 + 31 + 32 =132
And speaking of the factors 11, 2, 3, a nice palindromic expression for 132 is 11*2*3+3*2*11
132 is a Harshad (Joy-Giver) number, since it is divisible by the sum of its digits.
132 is also a self number, as there is no number n which added to the sum of the digits of n is equal to 132.
132 is not a palindrome in any base 2-12, but in base 7(246) it has digits that are each the double of the digits in 132. (I just noticed that, and wonder how often something like that happens?)
If the rods are placed in a circular arrangement instead of linear, then a correct solution will involve always moving the smallest disk one rod clockwise every other move. The alternate moves are forced.
1364 Founding of the Uniwersytet Jagiellonski in Krakow,
King Casimir III of Poland received permission to found an institution of higher learning (first called Krakow Academy)in Poland from Pope Urban V. A royal charter of foundation was issued on 12 May 1364, and a simultaneous document was issued by the City Council granting privileges to the Studium Generale. The King provided funding for one chair in liberal arts, two in Medicine, three in Canon Law and five in Roman Law, funded by a quarterly payment taken from the proceeds of the royal monopoly on the salt mines at Wieliczka.
Copernicus (1473-1543) was a student in 1491‑1496 (or 1495) and there is a statue in the library courtyard.
The University of Bologna is the oldest university in Europe and at the beginning of the eighteenth century students were still examined by public disputation, i.e. the candidate was expected to orally defend a series of academic theses. At the beginning of 1732 Bassi took part in a private disputation in her home with members of the university faculty in the presence of many leading members of Bolognese intellectual society. As a result of her performance during this disputation she was elected a member of the prestigious Bologna Academy of Science on 20th March. Rumours of this extraordinary young lady quickly spread and on 17th April she defended forty-nine theses in a highly spectacular public disputation. On 12th May following a public outcry she was awarded a doctorate from the university in a grand ceremony in the city hall of Bologna. Following a further public disputation the City Senate appointed her professor of philosophy at the university, making her the first ever female professor at a European university.See more at *Thony Christie, The Renaissance Mathematicus
1796 A paper on “Newton's Binomial Theorem Legally Demonstrated by Algebra” read to the Royal Society by the Rev. William Sewell, A. M. Communicated by Sir Joseph Banks, Bart. K. B. P. R. S.
1819 Sophie Germain penned a letter from her Parisian home to Gauss in which she gave a strategy for a general proof of Fermat’s last theorem. Germain's letter to Gauss contained the first substantial progress toward a proof in 200 years. *WIK "... I have never ceased to think of the theory of numbers. ... A long time before our Academy proposed as the subject of a prize the proof of the impossibility of Fermat's equation, this challenge ... has often tormented me." *MacTutor
"In this letter she laid out her grand plan to prove Fermat's Last Theorem. Her goal was to prove that for each odd prime exponent p, there are an infinite number of auxiliary primes of the form 2Np+1 such that the set of non-zero p-th power residues x^p mod (2Np+1) does not contain any consecutive integers. If there were a solution to x^p + y^p = z^p, then Germain observes that any such auxiliary prime would have to necessarily divide one of the numbers x, y, or z. Her letter and manuscripts found in various libraries showed her analysis for the primes p less than 100 and for auxiliary primes with N from 1 to 10." *Larry Riddle
Department of Mathematics, Agnes Scott College
1930, the Adler Planetarium and Astronomical Museum was opened to the public in Chicago, Illinois. A program using the Zeiss II star projector was presented by Prof. Philip Fox, who resigned from the staff of Northwestern Observatory to take charge of the new $1 million facility. Housed in a granite building, it was donated to the city by Max Adler, retired vice president of Sears, Roebuck & Co. He had been so impressed when he previously visited the world’s first planetarium at the Deutsches Museum, Munich, Germany, that he resolved to construct America's first modern planetarium open to the public in his home city. Its site was within the fairgrounds of the Century of Progress Exposition in 1933-34, and was an outstanding attraction. *TIS
1936, the Dvorak typewriter keyboard was patented in the U.S. by Dvorak and Dealey (Patent No. 2,040,248). The efficiency experts August Dvorak (a cousin of the composer) and William Dealey studied the typewriter to determine that they could arrange the keys in a new way which would speed up the operators of the typewriter. They designed a keyboard to maximize efficiency by placing common letters on the home row, and make the stronger fingers of the hands do most of the work. By contrast, the original QWERTY layout was designed for the earlier, less efficient typewriters. Previously, speed would result in two type bars hitting each other in their travel, so the original keyboard was laid out to reduce collisions.
Michael Will wrote, "And yet, here we are, 88 years later and all Qwerty. A testament to the power and plasticity of the human brain and hands. Typing is probably the best high school class I took."
"James Burke always showed how the great advances in science & tech weren't always from brilliant theory. Rather, they often came from simple crossovers between skill sets."
My response was to remark on the fact that just as public schools were starting tp push programming and computer skills classes, they took away typing classes.
1941 Zuse Completes Z3 Machine:
Konrad Zuse completes his Z3 computer, the first program-controlled electromechanical digital computer. It followed in the footsteps of the Z1 - the world’s first binary digital computer - which Zuse had developed in 1938. Much of Zuse’s work was destroyed in World War II, although the Z4, the most sophisticated of his creations, survives. *CHM For a little more information and perspective on Zuse and his creations, see this Renaissance Mathematicus blog.
1984 The Hindu newspaper from Madras, India, reported the unveiling of a statue of Srinivasa Ramanujan. [Mathematics Magazine 57 (1984), p 244]. *VFR
2004 discovery of what was believed to be the world's oldest seat of learning, the Library of Alexandria, was announced by Zahi Hawass, president of Egypt's Supreme Council of Antiquities during a conference at the University of California. A Polish-Egyptian team had uncovered 13 lecture halls featuring an elevated podium for the lecturer. Such a complex of lecture halls had never before been found on any Mediterranean Greco-Roman site. Alexandria may be regarded as the birthplace of western science, where Euclid discovered the rules of geometry, Eratosthenes measured the diameter of the Earth and Ptolemy wrote the Almagest, the most influential scientific book about the nature of the Universe for 1,500 years*TIS
 |
2013, This is the third "Pythagorean Day" of the 21st Century, 5/12/13. The first was on March 4, 2005 (3/4/05) and the second on June 8, 2010. How many more will there be in the 21st Century, and when is the next one?
1820 Florence Nightingale (12 May 1820 – 13 August 1910) is remembered as the mother of modern nursing. But few realize that her place in history is at least partly
linked to her use, following William Farr, Playfair and others, of graphical methods to convey complex statistical information dramatically to a broad audience. An example of "Stigler's Law of Eponomy" (Stigler, 1980), Nightingale's Coxcomb chart did not orignate with her, though this should not detract from her credit. She likely got the idea from William Farr, a close friend and frequent correspondent, who used the same graphic principles in 1852. The earliest known inventor of polar area charts is Andre-Michel Guerry (1829). [gallery of data visualization]
Pearson wrote of here, "Her statistics were more than a study, they were indeed her religion. For her Quetelet was the hero as scientist, and the presentation copy of his Physique sociale is annotated by her on every page. ... she held that the universe -- including human communities -- was evolving in accordance with a divine plan; that it was man's business to endeavor to understand this plan and guide his actions in sympathy with it. But to understand God's thoughts, she held we must study statistics, for these are the measure of His purpose. Thus the study of statistics was for her a religious duty.
K Pearson, The Life, Letters and Labours for Francis Galton (1924). *SAU
1845 Henri Brocard (12 May 1845 – 16 January 1922) who published (1897–99) a two volume catalog of plane curves and their properties. *VFR
His best-known achievement is the invention and discovery of the properties of the Brocard points, the Brocard circle, and the Brocard triangle, all bearing his name. Contemporary mathematician Nathan Court wrote that he, along with Émile Lemoine and Joseph Neuberg , was one of the three co-founders of modern triangle geometry.
In a triangle ABC with sides a, b, and c, where the vertices are labeled A, B and C in counterclockwise order, there is exactly one point P such that the line segments AP, BP, and CP form the same angle, ω, with the respective sides c, a, and b, namely that
*Wik
1851 Samuel Dickstein (May 12, 1851 – September 29, 1939) was a Polish mathematician of Jewish origin. He was one of the founders of the Jewish party "Zjednoczenie" (Unification), which advocated the assimilation of Polish Jews.
He was born in Warsaw and was killed there by a German bomb at the beginning of World War II. All the members of his family were killed during the Holocaust.
Dickstein wrote many mathematical books and founded the journal Wiadomości Mathematyczne (Mathematical News), now published by the Polish Mathematical Society. He was a bridge between the times of Cauchy and Poincaré and those of the Lwów School of Mathematics. He was also thanked by Alexander Macfarlane for contributing to the Bibliography of Quaternions (1904) published by the Quaternion Society.
He was also one of the personalities, who contributed to the foundation of the Warsaw Public Library in 1907.*Wik
1857 Oskar Bolza (12 May 1857–5 July 1942) After studying with Weierstrass and Klein, and realizing the difficulties of obtaining a suitable position in Germany, he came to the U.S. where he played an important role in the development of mathematics at Hopkins, Clark and Chicago. *VFR He published "The elliptic s-functions considered as a special case of the hyperelliptic s-functions" in 1900. From 1910, he worked on the calculus of variations. Bolza wrote a classic textbook on the subject, "Lectures on the Calculus of Variations" (1904). He returned to Germany in 1910, where he researched function theory, integral equations and the calculus of variations. In 1913, he published a paper presenting a new type of variational problem now called "the problem of Bolza." The next year, he wrote about variations for an integral problem involving inequalities, which later become important in control theory. Bolza ceased his mathematical research work at the outbreak of WW I in 1914.*TIS
1902 Frank Yates FRS (May 12, 1902 – June 17, 1994) was one of the pioneers of 20th century statistics. In 1931 Yates was appointed assistant statistician at Rothamsted Experimental Station by R.A. Fisher. In 1933 he became head of statistics when Fisher went to University College London. At Rothamsted he worked on the design of experiments, including contributions to the theory of analysis of variance and originating Yates' algorithm and the balanced incomplete block design. During World War II he worked on what would later be called operational research. *Wikipedia
1910 Dorothy Mary Hodgkin OM FRS (12 May 1910 – 29 July 1994), known professionally as Dorothy Crowfoot Hodgkin or simply Dorothy Hodgkin, was a British biochemist who developed protein crystallography, for which she won the Nobel Prize in Chemistry in 1964.
She advanced the technique of X-ray crystallography, a method used to determine the three-dimensional structures of biomolecules. Among her most influential discoveries are the confirmation of the structure of penicillin that Ernst Boris Chain and Edward Abraham had previously surmised, and then the structure of vitamin B12, for which she became the third woman to win the Nobel Prize in Chemistry.
In 1969, after 35 years of work and five years after winning the Nobel Prize, Hodgkin was able to decipher the structure of insulin. X-ray crystallography became a widely used tool and was critical in later determining the structures of many biological molecules where knowledge of structure is critical to an understanding of function. She is regarded as one of the pioneer scientists in the field of X-ray crystallography studies of biomolecules. *Wik
A three dimensional contour map of the electron density of penicillin derived from x-ray diffraction. The points of highest density show the positions of individual atoms in the penicillin. This device was used by Hodgkin to deduce the structure.
1919 Wu Wenjun (Chinese: 吴文俊; 12 May 1919 – 7 May 2017), also commonly known as Wu Wen-tsün, was a Chinese mathematician, historian, and writer. He was an academician at the Chinese Academy of Sciences (CAS), best known for Wu class, Wu formula, and Wu's method of characteristic set.
1926 James Samuel Coleman (May 12, 1926 – March 25, 1995) was a U.S. sociologist, a pioneer in mathematical sociology whose studies strongly influenced education policy. In the early 1950s, he was as a chemical engineer with Eastman-Kodak Co. in Rochester, N.Y. He then changed direction, fascinated with sociology and social problems. In 1966, he presented a report to the U.S. Congress which concluded that poor black children did better academically in integrated, middle-class schools. His findings provided the sociological underpinnings for widespread busing of students to achieve racial balance in schools. In 1975, Coleman rescinded his support of busing, concluding that it had encouraged the deterioration of public schools by encouraging white flight to avoid integration.*TIS
1977 Maryam Mirzakhani (12 May 1977 – 14 July 2017) was an Iranian mathematician and a professor of mathematics at Stanford University. Her research topics included Teichmüller theory, hyperbolic geometry, ergodic theory, and symplectic geometry. In 2005, as a result of her research, she was honored in Popular Science's fourth annual "Brilliant 10" in which she was acknowledged as one of the top 10 young minds who have pushed their fields in innovative directions.
Both Maryam Mirzakhani and her friend Roya Beheshti made the Iranian Mathematical Olympiad team in 1994. The international competition was held that year in Hong Kong and Mirzakhani scored 41 out of 42 and was awarded a gold medal. Beheshti was awarded a silver medal. Again in 1995 Mirzakhani was a member of the Iranian Mathematical Olympiad team. This time the international competition was held in Toronto, Canada, and Mirzakhani scored 42 out of 42 and was again awarded a gold medal.
On 13 August 2014, Mirzakhani was honored with the Fields Medal, the most prestigious award in mathematics, becoming the first Iranian to be honored with the award and the first of only two women to date. The award committee cited her work in "the dynamics and geometry of Riemann surfaces and their moduli spaces".
On 14 July 2017, Mirzakhani died of breast cancer at the age of 40
*Wik, *MacTutor
 |
| Maryam, (+ epsilon) with the other Field's Medalist of 2014 |
DEATHS
1003 Gerbert d'Aurillac (Pope Sylvester II) (c. 946 – 12 May 1003) French scholar who reintroduced the use of the abacus in mathematical calculations. He may have adopted the use of Arabic numerals (without the zero) from Khwarizmi. He built clocks, organs and astronomical instruments based on translations of Arabic works(One of his mechanical instruments was an oracular metal cast head that answered questions yes or no, sort of a tenth century magic 8-ball with speaking ability). (He was often accused after his death of being in league with demons )
He made no original contribution to mathematics or astronomy . However, he served in the all-important role of popularizer, communicating the value and importance of science to the uninitiated public. With the inspiration of Gerbert, Europe began its slow crawl out of the Dark Ages.*TIS
Sylvester, in blue, as depicted in the Gospels of Otto III
1682 Michelangelo Ricci ( 30 Jan., 1619; Rome, - 12 May, 1682; Rome) was a friend of Torricelli; in fact both were taught by Benedetti Castelli. He studied theology and law in Rome and at this time he became friends with René de Sluze. It is clear that Sluze, Torricelli and Ricci had a considerable influence on each other in the mathematics which they studied.
Ricci made his career in the Church. His income came from the Church, certainly from 1650 he received such funds, but perhaps surprisingly he was never ordained. Ricci served the Pope in several different roles before being made a cardinal by Pope Innocent XI in 1681.
Ricci's main work was Exercitatio geometrica, De maximis et minimis (1666) which was later reprinted as an appendix to Nicolaus Mercator's Logarithmo-technia (1668). It only consisted of 19 pages and it is remarkable that his high reputation rests solely on such a short publication.
In this work Ricci finds the maximum of xm(a - x)n and the tangents to ym = kxn. The methods are early examples of induction. He also studied spirals (1644), generalised cycloids (1674) and states explicitly that finding tangents and finding areas are inverse operations (1668). *SAU
In his own time Ricci's fame as a mathematician rested more on the many letters he wrote on mathematical topics, rather than on his published work. He corresponded with many mathematicians across Europe including Clavius, Viviani and de Sluze.
1684 Edme Mariotte(1620 ? – 12 May 1684) Little is known about his early life in the Cote d'Or region of eastern France, but in 1660 he discovered the eye's blind spot.and supposedly amazed the French Royal Court. At this time he may have been working at a Parish Church, but that is not known. In 1668 Colbert invited Mariotte to participate in the "l'Académie des Sciences", and in 1670 he moved to Paris. He published regularly right from his appointment. He is actually pictured in the portrait of the Establishment of the Academy, just to the left of Huygens and Cassini (he is sixth from the right in the picture).
 |
| *Wikipedia |
The first volume of the Academies papers was released in 1673, and he had many of the articles. His scope reached across the natural sciences including papers on fluid motion, heat, sound and acoustics, air pressure, and freezing water. When he is known at all, it is usually as confirming what we now call Boyle's Law, but in fact his work went well beyond what Hooke and Boyle had shown, and he demonstrated that the pressure decreased in arithmetic progression as the altitude changed in geometric progression. He also was the first to explain how the altitude at a high place could be calculated with a barometer. He did not give a formula, but described a procedure assuming that a rise of 63 "Paris feet" resulted in the drop in the barometric reading of 1 line or 1/144th of an inch. And I choose to call the desk toy called Newton's cradle by so many, Mariotte's cradle, since he was the first to describe this law of impact between bodies. Edme quit the Academy in 1681 and died on 12 May 1684 in Paris.
1742 Joseph Privat de Molières (1677 in Tarascon, Bouches-du-Rhône, France - 12 May 1742 in Paris, France) In 1723 he was appointed to a chair at the Collège Royal to succeed Varignon.
He argued against Newton and for Descartes' view of physics although he knew Newton's to be the more precise. Of course, although we now accept Newton's ideas of gravitation without much thought, it is clear if one thinks about it for a while that the idea of action at a distance through a vacuum is absurd. Many around this time voiced such an opinion (Newton himself realised this was a weakness in his theories) but where Privat de Molières differed from other critics of Newton's theory of gravitation is that he attempted to make a mathematically sound theory based on the idea of vortices. Understanding the accuracy of the theory of gravitation, Privat attempted to bring Newton's calculations into the vortex theory of matter of Malebranche. The problem was Kepler's laws, easily explained by Newton, but the cause of real problems for Descartes' vortex theory of planetary motion. In fact in a memoir written in 1733 Privat criticised Newton's theories for being too accurate saying that physical phenomena did not have geometrical precision *SAU
1753 Nicolas Fatio de Duillier (alternative names are Facio or Faccio;) (26 February 1664 – 12 May 1753) was a Swiss mathematician known for his work on the zodiacal light problem, for his very close (some have suggested "romantic" ) relationship with Isaac Newton, for his role in the Newton v. Leibniz calculus controversy , and for originating the "push" or "shadow" theory of gravitation.
[Le Sage's theory of gravitation is a kinetic theory of gravity originally proposed by Nicolas Fatio de Duillier in 1690 and later by Georges-Louis Le Sage in 1748. The theory proposed a mechanical explanation for Newton's gravitational force in terms of streams of tiny unseen particles (which Le Sage called ultra-mundane corpuscles) impacting all material objects from all directions. According to this model, any two material bodies partially shield each other from the impinging corpuscles, resulting in a net imbalance in the pressure exerted by the impact of corpuscles on the bodies, tending to drive the bodies together.]
He also developed and patented a method of perforating jewels for use in clocks.
When Leibniz sent a set of problems for solution to England he mentioned Newton and failed to mention Faccio among those probably capable of solving them. Faccio retorted by sneering at Leibniz as the ‘second inventor’ of the calculus in a tract entitled ‘Lineæ brevissimæ descensus investigatio geometrica duplex, cui addita est investigatio geometrica solidi rotundi in quo minima fiat resistentia,’ 4to, London, 1699. Finally he stirred up the whole Royal Society to take a part in the dispute (Brewster, Memoirs of Sir I. Newton, 2nd edit. ii. 1–5).
In 1707, Fatio came under the influence of a fanatical religious sect, the Camisards, which ruined Fatio's reputation. He left England and took part in pilgrim journeys across Europe. After his return only a few scientific documents by him appeared. He died in 1753 in Maddersfield near Worcester, England. After his death his Geneva compatriot Georges-Louis Le Sage tried to purchase the scientific papers of Fatio. These papers together with Le Sage's are now in the Library of the University of Geneva.
Eventually he retired to Worcester, where he formed some congenial friendships, and busied himself with scientific pursuits, alchemy, and the mysteries of the cabbala. In 1732 he endeavoured, but it is thought unsuccessfully, to obtain through the influence of John Conduitt [q. v.], Newton's nephew, some reward for having saved the life of the Prince of Orange. He assisted Conduitt in planning the design, and writing the inscription for Newton's monument in Westminster Abbey. *Wik
1856 Jacques Philippe Marie Binet (February 2, 1786 – May 12, 1856) was a French mathematician, physicist and astronomer born in Rennes; he died in Paris, France, in 1856. He made significant contributions to number theory, and the mathematical foundations of matrix algebra which would later lead to important contributions by Cayley and others. In his memoir on the theory of the conjugate axis and of the moment of inertia of bodies he enumerated the principle now known as Binet's theorem. He is also recognized as the first to describe the rule for multiplying matrices in 1812, and Binet's formula expressing Fibonacci numbers in closed form is named in his honour, although the same result was known to Abraham de Moivre a century earlier.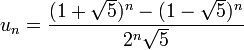
*Wik
Cauchy wrote his obituary, the only one he ever wrote. Apparently Cauchy was motivated by their common Bourbon fervour. [Ivor Grattan-Guiness, Convolutions in French Mathematics, 1800–1840, p. 192] *VFR
1859 Robert Leslie Ellis (25 August 1817 – 12 May 1859) was an English polymath, remembered principally as a mathematician and editor of the works of Francis Bacon. A brilliant man with broad interests and abilities who suffered from ill health all his short life. Senior Wrangler in the Mathematical tripos at Cambridge and also First Smith's prizeman. In 1840 he became a fellow of Trinity College and was interested in areas of mathematics which involved philosophical ideas. *SAU
1910 Sir William Huggins (7 Feb 1824; 12 May 1910 at age 86) English astronomer who explored the spectra of stars, nebulae and comets to interpret their chemical composition, assisted by his wife Margaret Lindsay Murray. He was the first to demonstrate (1864) that whereas some nebulae are clusters of stars (with stellar spectral characteristics, ex. Andromeda), certain other nebulae are uniformly gaseous as shown by their pure emission spectra (ex. the great nebula in Orion). He made spectral observations of a nova (1866). He also was first to attempt to measure a star's radial velocity. He was one of the wealthy 19th century private astronomers that supported their own passion while making significant contributions. At age only 30, Huggins built his own observatory at Tulse Hill, outside London *TIS
1952 George Lidstone (11 Dec 1870 in London, England - 12 May 1952 in Edinburgh, Scotland) was an actuary who worked for various Edinburgh insurance companies. He wrote papers on various numerical and statistical topics. *SAU Actuary in the modern sense originated in the middle of the 19th Century. Before that, the term was used for a court clerk. the term is from the Latin for bookkeeper.
Credits :
*CHM=Computer History Museum
*FFF=Kane, Famous First Facts
*NSEC= NASA Solar Eclipse Calendar
*RMAT= The Renaissance Mathematicus, Thony Christie
*SAU=St Andrews Univ. Math History
*TIA = Today in Astronomy
*TIS= Today in Science History
*VFR = V Frederick Rickey, USMA
*Wik = Wikipedia
*WM = Women of Mathematics, Grinstein & Campbell