On This Day in Math - February 29

 

MONTESSORI GIVING A LESSON IN TOUCHING GEOMETRICAL INSET

Common sense is not really so common.

~Antoine Arnauld (1612 - 1694) in
The Art of Thinking: Port-Royal Logic

The 60th day of the year; 60 is the smallest composite number which is the order of a simple group.

7! is the smallest # with 60 divisors.

There are four Archimedean solids with 60 vertices , : the truncated icosahedron, (shown) the rhombicosidodecahedron, the snub dodecahedron, and the truncated dodecahedron.

Oh, and Pi day is coming up in a couple of weeks, so ... suppose you were scrolling through the digits of pi and wondered how long it would take until you found a string of ten digits that had all ten of 0 through nine in it... Benjamin Vitale ‏@BenVitale thought to find out and :

You can arrange the whole numbers from 1 to 60 into pairs so that the sum of the numbers in each pair is a perfect square; in fact, you can do it in   4,366,714 ways. Here is one of those presented in a pretty fashion using only five squares for the sums. *Gordon Hamilton, Kiran S. Kedlaya, and Henri Picciotto; Square–Sum Pair Partitions(Won the George Polya Prize from MAA for 2016)

EVENTS

---Leap day is also called “bissextile” or “second sixth.” Under the Julian Calendar the leap day was added just before February 25, more specifically “ante diem sexto Kalendas Martius” (sixth day before the Calends of March) and was called “bissexto Kalendas Martius” (the second-six of the Calends of March). [Scientific American] *VFR

---

1688  On Feb. 29, 1688, Robert Hooke gave a lecture to the Royal Society of London about geological changes that the earth had undergone in its long history. It was one in a series of lectures on geology that Hooke gave over a 30-year span, and which were later printed as "Discourse of Earthquakes" in his Posthumous Works (1705). But this lecture was special, for here Hooke called attention to the importance of fossils in working out the earth's geological history. Shells found in rocks, says Hooke, are "… Records of Antiquity which Nature have left as Monuments and Hieroglyphick Characters of preceding Transactions in the ... Body of the Earth, which are infinitely more evident and certain tokens than any thing of antiquity than can be fetched out of Coins or Medals." Since most of his contemporaries considered fossils to be figured stones that grew in the earth, or lusus naturae, jokes of nature put there to amuse us, Hooke's pronouncement was a remarkable statement, a declaration of his belief that fossils are the remains of once living creatures, and that their location in rocks has to be explained, even if it means turning land into sea and sea into land. His comparison to ancient coins is a wonderful analogy; if we can reconstruct the history of Roman Britain from coins that we find in ruins, why can we not reconstruct the history of the earth from its coins and medals, the fossils in the rocks? *Linda Hall Org

---

1712 Sweden briefly had a February 30. In planning to switch from the Julian to the Gregorian calendar, the Swedish Empire resolved to omit leap days from 1700 to 1740. It followed through on this plan in 1700, but through error 1704 and 1708 remained leap years. With the time now out of joint, the empire abandoned its plan and returned to the Julian calendar by observing two leap days, February 29 and February 30, in 1712. (Sweden finally converted to the Gregorian calendar in 1753.)
If the original plan had been carried out, a person born on Feb. 29, 1696, would not celebrate a birthday until 1744. As it was, a person born on Feb. 30, 1712, would never celebrate a birthday at all. *Greg Ross, Futility Closet

February 30 existed from 1930–1931 after the Soviet Union introduced a revolutionary calendar in 1929. This calendar featured five-day weeks, 30-day months for every working month, and the remaining five or six days were “monthless” holidays. The abolition of the seven-day week in favor of a five-day week was intended to improve industrial efficiency by avoiding the regular interruption of a non-working day.

---

1504 On Feb. 29, 1504, Columbus and his men had been shipwrecked in Jamaica for eight months, relying on the local natives to feed them. After six months, the natives cut off the charity. Columbus, who had an astronomical almanac with him, summoned the native leaders and warned that if the natives did not reconsider, God would make the Moon disappear. When, as Columbus had predicted, the Moon became fully eclipsed that night, the natives panicked. *http://listosaur.com (Somewhat skeptical of this story. Did astronomical almanacs this early already include information like this about Jamaica?  And since lunar eclipses happen once or twice a year, wouldn't most of these leaders have witnessed more than one?)

Mark Twain seems to have known of this episode, and used it as a basis for a segment of his 1889 novel A Connecticut Yankee in King Arthur's Court. Reversing the Shawnee episode Twain has Hank Morgan, the Yankee in the title (and Bing Crosby in the movie version), hoodwinking the ignorant folk in King Arthur's England by invoking prior knowledge of a solar eclipse due in June of AD 528, even stating the precise time of totality. He used his imagination, though: there was no eclipse around that epoch.

---

1880 On Leap Day in 2012, John D Cook at The Endeavor blog posted a tribute from Pirates of Penzance by Gilbert & Sullivan :

"For some ridiculous reason, to which, however, I’ve no desire to be disloyal,
Some person in authority, I don’t know who, very likely the Astronomer Royal,
Has decided that, although for such a beastly month as February, twenty-eight days as a general rule are plenty,
One year in every four, its days shall be reckoned as nine and twenty."

---

In 1916, Maria Montessori of Rome, Italy, received a U.S. patent for “Cut-Out Geometrical Figure for Didactical Purposes” (No. 1,173,298). Montessori is famous for her educational methods. The patent covered her invention of a plate with recesses into which geometrical shapes can be fitted so the “fundamental principles of geometry may be easily and rapidly taught to young pupils.” The recesses can be filled with, for example, several smaller triangles that fill the larger triangular cavity.*TIS

 

---

1924 The first Josiah Willard Gibbs lecture was delivered by Professor M. I. Pupin of Columbia University to an AMS meeting in New York City. In introducing him, AMS president Veblen said “It is hoped that the Willard Gibbs Lecturers will remind the mathematicians of something that we fear they sometimes forget, – the existence of an outside world. It is equally hoped that they will remind the outside world that mathematics is a growing concern, – not a pedantic exercise for the torment of schoolboys, but a living organism growing larger and stronger each year.” See BAMS 30(1924), p. 289. *VFR

---

In 1936, Nature carried Niels Bohr's “bowl of balls” explanation for the effect of bombarding particles on a nucleus (Vol. 137, p. 344). He followed up in an article in Science (20 Aug 1937, p. 161) explaining that “to understand the typical features of nuclear transmutations initiated by impacts of material particles... A simple mechanical model which illustrates these features of nuclear collisions is ... a shallow basic with a number of billard balls in it. If the bowl were empty, then a ball which was sent in would go down one clope and pass out on the opposite side with its original energy. When, however, there are other balls in the bowl, then the incident one will not be able to pass through freely but will divide its energy first with one of the balls, these two will share their energy ... divided among all the balls.”*TIS

Suspended In Language: Niels Bohrs Life, Discoveries, And The Century He Shaped
by Jim Ottaviani, Leland Purvis and Jeff Parker

---

1940  Ernest O. Lawrence delivered his 1939 Nobel Prize in Physics banquet speech in Berkeley, California, having arranged to receive the prize there, rather than Sweden, so he would not lose time away from the task of fund raising for his cyclotron research. He was awarded the prize “for the invention and development of the cyclotron and for results obtained with it, especially with regard to artificial radioactive elements.” Lawrence said the discoveries made had “immediate practical significance” as “the new radiations and radioactive substances have opened vistas for all the sciences, especially in medical research and therapy.” He looked forward to a new research frontier using energies above a hundred million volts with “a giant cyclotron perhaps weighing more than 4,000 tons - twenty times larger.”«

---

1968 British astronomer, Jocelyn Burnell, announced the discovery of a pulsar, a pulsating radio source believed to be a rapidly rotating neutron star. *VFR

---

BIRTHS

1804 Antoine Hercule Romuald Florence ( 29 Feb 1804; 27 Mar 1879) was a French inventor, photographer and printer who coined the word “photographie” in French (before the use of “photography” in English suggested by John Herschel). Florence pioneered a process of reproducing drawings on paper sensitized with silver salts. He developed his ideas from 1832, before the better known Louis Daguerre. He was then living in Brazil, where he had settled in 1824 after working on board ships. During 1825-30, he had joined a scientific expedition as an illustrator and topographic draftsman. By 1832, his goal was document reproduction (in publications as illustrations) rather than portraits. He never published his discoveries to the larger scientific world in Europe, and his name was forgotten in history. In 1976, a Brazilian historian, Boris Kossoy, uncovered Florence's work, and studied his notes. Kossoy presented the results of his research at a photography symposium in 1976, and in a book published in 1980.*TIS

Photocopy of a diploma made using Florence's photographic technique, ca. 1833

---

1840  John Phillip Holland (29 Feb 1840; 12 Aug 1914 )Irish inventor known as the “father of the modern submarine,” who designed and built the first underwater vessel accepted by the U.S. Navy. In 1873, he emigrated to the U.S. where, with financial support from the Irish Fenian Society (who hoped to use submarines against England), he built the Fenian Ram, a small sub that proved a limited success in a test run. In 1895, his J.P. Holland Torpedo Boat Company received a contract from the U.S. Navy to build a submarine, and in 1898 a successful Holland, the first truly practical submarine, was launched. The U.S. government ordered six more; similar orders came from England, Japan, and Russia. Holland’s final years were marked by litigation with his financial backers.*TIS

Holland stands in the hatch of a submarine.

---

1860 Herman Hollerith (29 Feb 1860, 17 Nov 1929) American inventor of a tabulating machine that was an important precursor of the electronic computer. For the 1890 U.S. census, he invented several punched-card machines to automate the sorting of data. The machine which read the cards used a pin going through a hole in the card to make an electrical connection with mercury placed beneath. The resulting electrical current activated a mechanical counter. It saved the United States 5 million dollars for the 1890 census by completing the analysis of the data in a fraction of the time it would have taken without it and with a smaller amount of  manpower than would have been necessary otherwise. In 1896, he formed the Tabulating Machine Company, a precursor of IBM. *TIS

---

1928 Caleb Vance Haynes, Jr (born February 29, 1928)) is an American archeologist and geologist who was active (1996-2004) in the effort to keep the remains of prehistoric Kennewick Man for academic purposes, rather than letting the Army Corps of Engineers repatriate them to a modern Indian tribe. Haynes was one of  eight scientists that sued on 16 Oct 1996 for the right to study the bones. He provided the court deciding the matter with arguments that because the bones had been carbon dated to be about 9,000 years old, the remains could not be a direct ancestor of any modern tribe. The court's judgment was that Kennewick Man did not meet the definition of a native American, as used in the Native American Graves and Protection Act. The almost complete skeleton of Kennewick Man, found beside the Columbia River in Kennewick, Washington (28 Jul 1996), is one of the very few specimens available to understand the peopling of America in that era, and offers great value to science.« *TIS

Haynes coined the term "black mat" for a layer of 10,000-year-old swamp soil seen in many North American archaeological studies.

Haynes was elected in 1990 to the National Academy of Sciences. From 1996 to 2004, Haynes worked to keep the Kennewick Man discovery available for science. Currently an emeritus Regents' professor at the University of Arizona, Haynes is still active in the School of Anthropology.*Wik

---

1932 Gene Howard Golub (February 29, 1932 – November 16, 2007), Fletcher Jones Professor of Computer Science (and, by courtesy, of Electrical Engineering) at Stanford University, was one of the preeminent numerical analysts of his generation.
Credits. *Wik

---

DEATHS

1744 John Theophile Desaguliers (12 Mar 1683, 29 Feb 1744 at age 60)French-English chaplain and physicist who studied at Oxford, became experimental assistant to Sir Isaac Newton. As curator at the Royal Society, his experimental lectures in mechanical philosophy and electricity (advocating, substantiating and popularizing the work of Isaac Newton) attracted a wide audience. In electricity, he coined the terms conductor and insulator. He repeated and extended the work of Stephen Gray in electricity. He proposed a scheme for heating vessels such as salt-boilers by steam instead of fire. He made inventions of his own, such as a planetarium, and improvements to machines, such as Thomas Savery's steam engine (by adding a safety valve, and using an internal water jet to condense the steam in the displacement chambers) and a ventilator at the House of Commons. He was a prolific author and translator. *TIS

---

1932 Giuseppe Vitali (26 August 1875 – 29 February 1932) was an Italian mathematician who worked in several branches of mathematical analysis. He was the first to give an example of a non-measurable subset of real numbers, see Vitali set. His covering theorem is a fundamental result in measure theory. He also proved several theorems concerning convergence of sequences of measurable and holomorphic functions. Vitali convergence theorem generalizes Lebesgue's dominated convergence theorem. Another theorem bearing his name gives a sufficient condition for the uniform convergence of a sequence of holomorphic functions on an open domain D⊂ℂ to a holomorphic function on D. This result has been generalized to normal families of meromorphic functions, holomorphic functions of several complex variables, and so on. *Wik

---

1960 Teiji Takagi (21 April 1875 in Kazuya Village (near Gifu), Japan - 29 Feb 1960 in Tokyo, Japan) Takagi worked on class field theory, building on Heinrich Weber's work.*SAU

He is  best known for proving the Takagi existence theorem in class field theory. The Blancmange curve, the graph of a nowhere-differentiable but uniformly continuous function, is also called the Takagi curve after his work on it.

He was also instrumental during World War II in the development of Japanese encryption systems.

---

Credits
*VFR = V Frederick Rickey, USMA
*TIS= Today in Science History
*Wik = Wikipedia
*SAU=St Andrews Univ. Math History
*CHM=Computer History Museum
*FFF=Kane, Famous First Facts

On This Day in Math - February 28

  

Well David, I have a lot of ideas and throw away the bad ones.
Upon being asked how he had so many good ideas by David Harker, his student.
— Linus Pauling

The 59th day of the year; 59 is the center prime number in a 3x3 prime magic square that has the smallest possible total for each row, column and diagonal, 177.
In 1913, English puzzle writer Henry Dudeney gave an order 3 prime magic square that used the number 1. Although is was commonly included as a prime then,  present day convention no longer considers it a prime.

59 divides the smallest composite Euclid number 13# + 1= 13*11*7*5*2 + 1 = 59*509  (the symbol for a primorial,  n#, means the product of all primes from n down to 2)Euclid used numbers of the form n#+1 in his proof  that there are an infinite number of primes.

And at the right is one of the 59 stellations of the icosahedron.

Now for some nice observations from Derek Orr@MathYearRound:
5^59 - 4^59 is prime.
4^59 - 3^59 is prime.
3^59 - 2^59 is prime.
The first 59 digits of 58^57 form a prime.

---

EVENTS

1678 In a letter to Robert Boyle, Isaac Newton explained his concept of ether. “I suppose that there is diffused through all places an ethereal substance capable of contraction and dilation, strongly elastic and, in a word, much like air in all respects, but far more subtil.” He thought it was in all bodies of matter, but "rarer in the pores than in free spaces." This he suspects is the cause of light being refracted towards the perpendicular. *Rigaud, Letters of Scientific men, vol. 2, p. 407

---

1695 Leibniz writes to Johann Bernoulli encouraging him to use the term calculus summatorus which Liebniz used for integration.

*VFR

---

1825 Cauchy presented to the Acad´emie a paper on integrals of complex-valued functions where the limits of integration were allowed to be complex. Previously, he had done much work on such
integrals when the limits were real. [Grattan-Guinness, 1990, p. 766] *VFR

---

1905 Lise Meitner had entered the University of Vienna in October 1901. She was particularly inspired by Boltzmann, and was said to often speak with contagious enthusiasm of his lectures. Her dissertation was supervised by Franz Exner and his assistant Hans Benndorf. Her thesis, titled Prüfung einer Formel Maxwells ("Examination of a Maxwell Formula"), was submitted on 20 November 1905 and approved on 28 November. She was examined orally by Exner and Boltzmann on 19 December, and her doctorate was awarded on 1 February 1906.

 She became the second woman to earn a doctoral degree in physics at the University of Vienna, after Olga Steindler who had received her degree in 1903; the third was Selma Freud, who worked in the same laboratory as Meitner, and received her doctorate later in 1906.Freud is also known as  founder of the first official Salvation Army corps in Vienna.

---

1928 Chandrasekhara Venkata Raman led experiments at the Indian Association for the Cultivation of Science with collaborators, including K. S. Krishnan, on the scattering of light, when he discovered what now is called the Raman effect. Raman would win the Nobel Prize for this work. He was the first (and still the only) Indian scientist to win the Prize while a citizen of India. He was the first Asian and first non-white to receive any Nobel Prize in the sciences. He broke down at the presentation, in his own words because, " I turned round and saw the British Union Jack under which I had been sitting and it was then that I realised that my poor country, India, did not even have a flag of her own - and it was this that triggered off my complete breakdown."

India celebrates National Science Day on 28 February of every year to commemorate the discovery. *Wik

---

1935  On this day in 1935, the opera: The Necklace of the Sun : A Mayan Drama by the number theorist Derrick Norman Lehmer had its premiere at the Scottish Rite Auditorium, Oakland. It was also performed in San Francisco.*SAU  This was his second opera, he wrote The Harvest in 1933.

---

1953 James Watson, from early on this Saturday, spent his time at the Cavendish Laboratory in Cambridge, shuffling cardboard cutout models of the molecules of the DNA bases: adenine (A), guanine (G), cytosine (C) and thymine(T). After a while, in a spark of ingenuity, he discovered their complementary pairing. He realized that A joined with T had a close resemblance to C joined with G, and that each pair could hold together with hydrogen bonds. Such pairs could also neatly fit like rungs meeting at right-angles between two anti-parallel helical sugar-phosphate backbones of DNA wound around a common axis. Such structure was consistent with the known X-ray diffraction pattern evidence. Each separated helix with its half of the pairs could form a template for reproducing the molecule. The secret of life First announcement by Francis Crick and James Watson that they had reached their conclusion about the double helix structure of the DNA molecule. Their paper, A Structure for Deoxyribose Nucleic Acid, was published in the 25 Apr 1953 issue of journal Nature. *TIS

DNA model built by Crick and Watson in 1953, on display in the Science Museum, London

---

1956 Jay Forrester at MIT is awarded a patent for his coincident current magnetic core memory. Forrester's invention, given Patent No. 2,736,880 for a "multicoordinate digital information storage device," became the standard memory device for digital computers until supplanted by solid state (semiconductor) RAM in the mid-1970s. *CHM

---

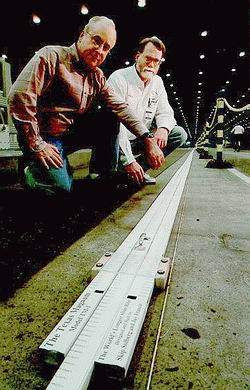
2001 With a length of 350 feet 6.6 inches and currently the World's Longest documented Slide Rule, The Texas Magnum by Skip Solberg and Jay Francis,was demonstrated on February 28, 2001 in the Lockeed-Martin Aircraft Assembly Facility at Air Force Plant 4 in Fort Worth, Texas. The Texas Magnum holds the world's record for the longest linear slide rule. The Texas Magnum was designed as a traditional Mannheim style slide rule. The A, C, D and L scales are included on the slide rule *International Slide Rule Museum

A little over two decades later I ran across the smallest slide rule I have ever seen in an Antique shop in Cadiz, Ky.  It was a sterling silver tie clip with a working center bar and slide, two inches long.

  And on a tip from Ted Courant, I found these even smaller cuff link working slide rules, 1.125" long

---

BIRTHS

1552 Joost Bürgi (28 Feb 1552, 31 Jan 1632) Swiss watchmaker and mathematician who invented logarithms independently of the Scottish mathematician John Napier. He was the most skilful, and the most famous, clockmaker of his day.  In clock-making circles, Burgi is renowned for inventing the cross-beat escapement. 

He also made astronomical and practical geometry instruments (notably the proportional compass and a triangulation instrument useful in surveying). This led to becoming an assistant to the German astronomer Johannes Kepler. Bürgi was a major contributor to the development of decimal fractions and exponential notation, but his most notable contribution was published in 1620 as a table of antilogarithms. Napier published his table of logarithms in 1614, but Bürgi had already compiled his table of logarithms at least 10 years before that, and perhaps as early as 1588. *TIS  For more in-depth look at Burgi's life, see this post at the Renaissance Mathematicus

astronomical clock by Burgi is in Stockholm. 

---

1704 Louis Godin (28 February 1704 Paris – 11 September 1760 Cadiz) was a French astronomer and member of the French Academy of Sciences. He worked in Peru, Spain, Portugal and France.
He was graduated at the College of Louis le Grand, and studied astronomy under Joseph-Nicolas Delisle. His astronomical tables (1724) gave him reputation, and the French Academy of Sciences elected him a pensionary member. He was commissioned to write a continuation of the history of the academy, left uncompleted by Bernard le Bovier de Fontenelle, and was also authorized to submit to the minister, Cardinal André-Hercule de Fleury, the best means of discovering the truth in regard to the figure of the earth, and proposed sending expeditions to the equator and the polar sea. The minister approved the plan and appropriated the necessary means, the academy designating Charles Marie de La Condamine, Pierre Bouguer, and Godin to go to Peru in 1734.
When they had finished their task in 1738, at the invitation of the Viceroy of Peru, Godin accepted the professorship in mathematics in Lima, where he also established a course of astronomical lectures. When in 1746 an earthquake destroyed the greater part of Lima, he took valuable seismological observations, assisted the sufferers, and made plans by the use of which the new buildings would be less exposed to danger from renewed shocks.
In 1751 he returned to Europe, but found that he had been nearly forgotten, and superseded as pensioner of the academy; and, as his fortune had been lost in unfortunate speculations, he accepted the presidency of the college for midshipmen in Cadiz in 1752. During the earthquake of Lisbon, 1755, which was distinctly felt at Cadiz, he took observations and did much to allay the apprehensions of the public, for which he was ennobled by the king of Spain. In 1759 he was called to Paris and reinstated as pensionary member of the academy, but he died on his return to Cadiz. *Wik

---

1735 Alexandre-Théophile Vandermonde (28 Feb 1735 in Paris, France - 1 Jan 1796 in Paris, France). was a French mathematician best known for his work on determinants. *SAU
In 1772 Vandermonde used [P]ⁿ to represent the product of the n factors p(p-1)(p-2)... (p-n+1). With such a notation [P]^p would represent what we would now write as p!, but I can imagine this becoming, over time, just [p] (De Morgan would do just such a thing in his 1838 essays on probability). Vandermonde seems to have been the first to consider [p]⁰ (or 0!) and determined it was (as we now do) equal to one. Vandermonde's notation included a method for skipping numbers, so that [p/3]ⁿ would indicate p(p-3)(p-6)... (p-3(n-1)). (this method seems better to me than the present method for factorials which skip terms) It even allowed for negative exponents. *PB

Vandermonde was a violinist, and became engaged with mathematics only around 1770. In Mémoire sur la résolution des équations (1771) he reported on symmetric functions and solution of cyclotomic polynomials; this paper anticipated later Galois theory. In Remarques sur des problèmes de situation (1771) he studied knight's tours, and presaged the development of knot theory by explicitly noting the importance of topological features when discussing the properties of knots:

"Whatever the twists and turns of a system of threads in space, one can always obtain an expression for the calculation of its dimensions, but this expression will be of little use in practice. The craftsman who fashions a braid, a net, or some knots will be concerned, not with questions of measurement, but with those of position: what he sees there is the manner in which the threads are interlaced"

---

1859 Florian Cajori (born 28 Feb 1859)Swiss-born U.S. educator and mathematician whose works on the history of mathematics were among the most eminent of his time.*TIS at times Cajori's work lacked the scholarship which one would expect of such an eminent scientist, we must not give too negative an impression of this important figure. He almost single-handedly created the history of mathematics as an academic subject in the United States and, particularly with his book on the history of mathematical notation, he is still one of the most quoted historians of mathematics today. *SAU

On my second copy of Cajori's A History of Mathematical Notation after wearing the first ragged.*PB

---

1878 Pierre Joseph Louis Fatou (28 Feb 1878 in Lorient, France - 10 Aug 1929 in Pornichet, France) was a French mathematician working in the field of complex analytic dynamics. He entered the École Normale Supérieure in Paris in 1898 to study mathematics and graduated in 1901 when he was appointed an astronomy post in the Paris Observatory. Fatou continued his mathematical explorations and studied iterative and recursive processes such as z == z²+C . the Julia set and the Fatou set are two complementary sets defined from a function.
Fatou wrote many papers developing a Fundamental theory of iteration in 1917, which he published in the December 1917 part of Comptes Rendus. His findings were very similar to those of Gaston Maurice Julia, who submitted a paper to the Académie des Sciences in Paris for their 1918 Grand Prix on the subject of iteration from a global point of view. Their work is now commonly referred to as the generalised Fatou–Julia theorem.*Wik  Fatou dust is a term applied to certain iteration sets that have zero area and an infinite number of disconnected components.

Julia sets for 𝑧^2+0.7885 𝑒^(𝑖𝑎) ,where a ranges from 0 to 2𝜋

*Wik

---

1901 Linus Carl Pauling (28 Feb 1901; 19 Aug 1994 at age 93) an American chemist, physicist and author who applied quantum mechanics to the study of molecular structures, particularly in connection with chemical bonding. Pauling was awarded the Nobel Prize for Chemistry in 1954 for charting the chemical underpinnings of life itself. Because of his work for nuclear peace, he received the Nobel Prize for Peace in 1962. He is remembered also for his strong belief in the health benefits of large doses of vitamin C.*TIS

---

1925 Louis Nirenberg (28 February 1925, Hamilton, Ontario, Canada - ) is a Canadian-born American mathematician, and one of the outstanding analysts of the twentieth century. He has made fundamental contributions to linear and nonlinear partial differential equations and their application to complex analysis and geometry.*Wik

---

1930 Leon N. Cooper (28 Feb 1930 - ) American physicist who shared (with John Bardeen and John Robert Schrieffer) the 1972 Nobel Prize in Physics, for his role in developing the BCS (for their initials) theory of superconductivity. The concept of Cooper electron pairs was named after him.*Wik

---

1939 Daniel C. Tsui (28 Feb 1939 - ) Chinese-American physicist who shared (with Horst L. Störmer and Robert B. Laughlin) received the 1998 Nobel Prize for Physics for the discovery and explanation that the electrons in a powerful magnetic field at very low temperatures can form a quantum fluid whose particles have fractional electric charges. This effect is known as the fractional quantum. *TIS

---

1948  Steven Chu FREng ForMemRS HonFInstP (born February 28, 1948) is an American physicist and former government official. He is a Nobel laureate and was the 12th U.S. secretary of energy. He is currently the William R. Kenan Jr. Professor of Physics and Professor of Molecular and Cellular Physiology at Stanford University. He is known for his research at the University of California, Berkeley, and his research at Bell Laboratories and Stanford University regarding the cooling and trapping of atoms with laser light, for which he shared the 1997 Nobel Prize in Physics with Claude Cohen-Tannoudji and William Daniel Phillips.

Chu served as U.S. Secretary of Energy under the administration of President Barack Obama from 2009 to 2013. At the time of his appointment as Energy Secretary, Chu was a professor of physics and molecular and cellular biology at the University of California, Berkeley, and the director of the Lawrence Berkeley National Laboratory, where his research was concerned primarily with the study of biological systems at the single molecule level. Chu resigned as energy secretary on April 22, 2013. He returned to Stanford as Professor of Physics and Professor of Molecular & Cellular Physiology

---

1954 Jean Bourgain(28 Feb 1954 - 22 Dec, 2018)Belgian mathematician who was awarded the Fields Medal in 1994 for his work in analysis. His achievements in several fields included the problem of determining how large a section of a Banach space of finite dimension n can be found that resembles a Hilbert subspace; a proof of Luis Antonio Santaló's inequality; a new approach to some problems in ergodic theory; results in harmonic analysis and classical operators; and nonlinear partial differential equations. Bourgain's work was noteworthy for the versatility it displayed in applying ideas from wide-ranging mathematical disciplines to the solution of diverse problems. *TIS

*Wik

---

DEATHS

1691 Joseph Moxon (8 August 1627 - February 1691 (Royal Society archives state his death date as 28 February; the Oxford Dictionary of National Biography states that he was buried on 15 February???{I hope one of them was wrong}), hydrographer to Charles II, was an English printer of mathematical books and maps, a maker of globes and mathematical instruments, and mathematical lexicographer. He produced the first English language dictionary devoted to mathematics, "Mathematicks made easie, or a mathematical dictionary, explaining the terms of art and difficult phrases used in arithmetick, geometry, astronomy, astrology, and other mathematical sciences". In November 1678, he became the first tradesman to be elected as a Fellow of the Royal Society. *Wik Thony Christie has written that he was one of the first English Printers to print tables of Logarithms.

---

1742 Willem's Gravesande (26 September 1688 – 28 February 1742)was a Dutch mathematician who expounded Newton's philosophy in Europe. In 1717 he became professor in physics and astronomy in Leiden, and introduced the works of his friend Newton in the Netherlands.
His main work is Physices elementa mathematica, experimentis confirmata, sive introductio ad philosophiam Newtonianam or Mathematical Elements of Natural Philosophy, Confirm'd by Experiments (Leiden 1720), in which he laid the foundations for teaching physics. Voltaire and Albrecht von Haller were in his audience, Frederic the Great invited him in 1737 to come to Berlin.
His chief contribution to physics involved an experiment in which brass balls were dropped with varying velocity onto a soft clay surface. His results were that a ball with twice the velocity of another would leave an indentation four times as deep, that three times the velocity yielded nine times the depth, and so on. He shared these results with Émilie du Châtelet, who subsequently corrected Newton's formula E = mv to E = mv². (Note that though we now add a factor of 1/2 to this formula to make it work with coherent systems of units, the formula as expressed is correct if you choose units to fit it.) *Wik

---

1863 Jakob Philipp Kulik (1 May 1793 in Lemberg, Austrian Empire (now Lviv, Ukraine) - 28 Feb 1863 in Prague, Czech Republic) Austrian mathematician known for his construction of a massive factor tables.
Kulik was born in Lemberg, which was part of the Austrian empire, and is now Lviv located in Ukraine.In 1825, Kulik mentioned a table of factors up to 30 millions, but this table no longer seems to exist. It is also not clear if it had really been completed.
From about 1825 until 1863 Kulik produced a factor table of numbers up to 100330200 (except for numbers divisible by 2, 3, or 5). This table basically had the same format that the table to 30 millions and it is therefore most likely that the work on the "Magnus canon divisorum" spanned from the mid 1820s to Kulik's death, at which time the tables were still unfinished. These tables fill eight volumes totaling 4212 pages, and are kept in the archives of the Academy of Sciences in Vienna. Volume II of the 8 volume set has been lost.*Wik

---

1956 Frigyes Riesz (22 Jan 1880; 28 Feb 1956) Hungarian mathematician and pioneer of functional analysis, which has found important applications to mathematical physics. His theorem, now called the Riesz-Fischer theorem, which he proved in 1907, is fundamental in the Fourier analysis of Hilbert space. It was the mathematical basis for proving that matrix mechanics and wave mechanics were equivalent. This is of fundamental importance in early quantum theory. His book Leçon's d'analyse fonctionnelle (written jointly with his student B Szökefalvi-Nagy) is one of the most readable accounts of functional analysis ever written. Beyond any mere abstraction for the sake of a structure theory, he was always turning back to the applications in some concrete and substantial situation. *TIS

---

2013 Donald A. Glaser (21 Sep 1926, 28 Feb 2013) American physicist, who was awarded the Nobel Prize for Physics in 1960 for his invention of the bubble chamber in which the behaviour of subatomic particles can be observed by the tracks they leave. A flash photograph records the particle's path. Glaser's chamber contains a superheated liquid maintained in a superheated, unstable state without boiling. A piston causing a rapid decrease in pressure creates a tendency to boil at the slightest disturbance in the liquid. Then any atomic particle passing through the chamber leaves a track of small gas bubbles caused by an instantaneous boiling along its path where the ions it creates act as bubble-development centers.*TIS  With the freedom that accompanies a Nobel Prize, he soon began to explore the new field of molecular biology, and in 1971 joined two friends, Ronald E. Cape and Peter Farley, to found the first biotechnology company, Cetus Corp., to exploit new discoveries for the benefit of medicine and agriculture. The company developed interleukin and interferon as cancer therapies, but was best known for producing a powerful genetic tool, the polymerase chain reaction, to amplify DNA. In 1991, Cetus was sold to Chiron Corp., now part of Novartis. Glaser died in his sleep Thursday morning, Feb. 28, at his home in Berkeley. He was 86. *Philosophy of Science Portal

---

1923 Freeman (John) Dyson (15 Dec 1923, Feb 28, 2020  ) is an English-born American physicist and educator best known for his speculative work on extraterrestrial civilizations. As an imaginative scientist he proposed that a highly advanced technological civilization would ultimately completely surround its host star with a huge shell to capture 100% of the useful radiant energy. This "Dyson shell", would have a gigantic cluster of artificial planetoids ("Dyson cloud") with billions of billions of inhabitants who would make use of the energy captured by the Dyson shell. He also made the intriguing speculation that a Dyson shell viewed from other galaxies would have a highly distinctive, unnatural light. He suggests astronomers search for such tell-tale colored stars, which should signify advanced, intelligent life. *TIS (One of Dyson's earliest memories of his calculating power was at a time when he was still being put down for naps. He set about summing the fractions 1+1/2 + 1/4 ... and realized that they added up to two. At a time when most of us were still trying to figure out what fractions were, Dyson summed an infinite converging sequence.)
I came across another beautiful anecdote about Dyson's incredible mental computational ability on the Math Frolic blog Posted by "Shecky Riemann":
Freeman Dyson sitting around a table with a bunch of scientists where the question arises, is there an integer such that by moving the last digit to the front (say 1234 to 4123) you can arrive at a result such that the new integer is exactly double the value of the original integer? In a matter of seconds, Dyson essentially responds (to a stunned group), “Oh, that’s not difficult, but of course the smallest such number is 18 digits long.” AND, he was right! If you don't want to track it down by yourself, the smaller number is at the bottom of this post.
He died in a Hospital near Princeton, where he was an emeritus professor.

---

1925 Cecilia Berdichevsky or Berdichevski (née Tuwjasz) (Mar 30, 1925 – Feb 28,2010) was a pioneering Argentine computer scientist and began her work in 1961 using the first Ferranti Mercury computer in that country.

She was born Mirjam Tuwjasz  in Vidzy, at that time part of Poland, now Belarus.

Because of growing hostilities toward the Jewish community,first her father and then her mother Hoda[2] and her emigrated to Argentina when she was four years old, where she adopted the name Cecilia, and she spent her childhood years in Avellaneda, south of the Buenos Aires suburbs. Her father died within a few years of arriving in their new home and her mother remarried a rich man.

Cecilia married Mario Berdichevsky, a physician from Avellaneda, in 1951. Despite having a good job as a practicing accountant for ten years, she was not happy there having experienced many frustrations. A friend, computer scientist Rebeca Guber, convinced her to go back to school, which changed her life.

At the age of 31, Berdichevsky began her studies of mathematics at the University of Buenos Aires with Manuel Sadosky. There she had her first experience programming the new Ferranti Mercury computer, which became known by the nickname "Clementina" after someone programmed it to play the American song, "My darling Clementine." In 1961, when it arrived in Buenos Aires from England, Clementina was the most powerful computer in the country, cost $300,000 and measured 18 metres (59 ft) in length. It was the first large computer used for scientific purposes in the country (in that same year, an IBM 1401 was installed in Buenos Aires for business uses).

The newly graduated Berdichevsky studied computing from the visiting English software engineer Cicely Popplewell (famous for having worked with Alan Turing in Manchester) and with the Spanish mathematician Ernesto García Camarero. A photoelectric device read a punched paper ribbon that was used to submit the data and Clementina produced the desired result in only seconds.

Berdichevsky worked with Sadosky's institute until an Argentine coup d'état that installed a military dictatorship, which imposed government control over the workings of the previously autonomous state universities. . Many academics, including Sadosky, were forced into exile.

In 1984, Berdichevsky became Deputy General Manager of the Argentine savings bank Caja de Ahorro in charge of its computer center. She was also named the representative at the International Federation for Information Processing.

After her retirement, she continued to work as a computer consultant and participated in important international projects and organizations such as United Nations Development Program.Cecilia Berdichevsky died in Avellaneda, Argentina, 28 February 2010

Typical paper tapes showing holes punched to input data to early computers.Both five hole and eight hole were common.

---

2010 Owen Chamberlain (July 10, 1920 – February 28, 2006) was an American physicist who shared with Emilio Segrè the Nobel Prize in Physics for the discovery of the antiproton, a sub-atomic antiparticle.

In 1948, having completed his experimental work, Chamberlain returned to Berkeley as a member of its faculty. There he, Segrè, and other physicists investigated proton-proton scattering. In 1955, a series of proton scattering experiments at Berkeley's Bevatron led to the discovery of the anti-proton, a particle like a proton but negatively charged. Chamberlain's later research work included the time projection chamber (TPC), and work at the Stanford Linear Accelerator Center (SLAC).

Chamberlain was politically active on issues of peace and social justice, and outspoken against the Vietnam War. He was a member of Scientists for Sakharov, Orlov, and Shcharansky, three physicists of the former Soviet Union imprisoned for their political beliefs. In the 1980s, he helped found the nuclear freeze movement. In 2003 he was one of 22 Nobel Laureates who signed the Humanist Manifesto.

Chamberlain was diagnosed with Parkinson's disease in 1985, and retired from teaching in 1989. He died of complications from the disease on February 28, 2006, in Berkeley at the age of 85. *Wik

---

Credits :
*CHM=Computer History Museum
*FFF=Kane, Famous First Facts
*NSEC= NASA Solar Eclipse Calendar
*RMAT= The Renaissance Mathematicus, Thony Christie
*SAU=St Andrews Univ. Math History
*TIA = Today in Astronomy
*TIS= Today in Science History
*VFR = V Frederick Rickey, USMA
*Wik = Wikipedia
*WM = Women of Mathematics, Grinstein & Campbell

I'm calling this Dyson's Number, 105,263,157,894,736,842