For those who may not be familiar with the term "cevian", it refers to a segment in a triangle from a vertex to the opposite side (extended if necessary). Angle bisectors, medians, and altitudes are all cevians then, but a perpendicular bisector of a side would not be because it doesn't necessarily pass through a vertex. The name is in honor of Giovanni Ceva and was originated in France in 1888 and has spread from there.
Professor Satterly seems to have created the term "nedians" as a comparison for medians to describe a cevian that cuts the opposite side 1/n th of the way from one vertex to the next. [I shall use the notation 4-nedian for a nedian that cuts 1/4th of the way along the opposite side,] A median would be the 2-nedian.
In the image, triangle ABC has 3-nedians AD, BE, and CF where D is 1/3 of the way from B to C; E is 1/3 of the way from C to A, etc.
The intersections of the three Nedians of a triangle will form another triangle at their three points of intersection, called the nedian triangle. [JKL in the image].
Professor Satterly seems to have discovered several properties of the nedians, and their nedian triangles which I will give here; and then I have come up with several interesting properties of my own about them that I will add to this blog.
It is not too difficult using affine properties of a triangle to verify many of these.
Professor Satterly showed that the sum of the squares of the nedians would be
Students may wish to explore these properties by creating Sketchpad or Geogebra interactive models to confirm them, and then challenge themselves to prove them. Proving them is easier with a property of affine geometry. Every triangle is affine equivalent to every other triangle in the plane. Under affine transformations areas and lengths may change, but ratios of them are preserved... which means that you can choose any triangle... a right triangle or an equilateral triangle, to find a property about ratios of lengths or areas, and it will apply to any other triangle... and that is a BIG idea to lock away (I am not well schooled in the particulars of affine geometry, so if I have mis-stated that in some way, please advise).
Professor Satterly also stated that the Nedian triangle will have an area of \(\frac{(n-2)^2}{n^2-n+1}\) the area of ABC. Note that for the median, or 2-nedian, the area diminishes to zero since the three medians intersect in a single point.
Professor Satterly suggested the term "backward nedian triangle" for a case in which the 1/n ratio went in the opposite order (Let D be 1/3 of the way from C to B insted). This can be eliminated if we simply allow any real number for the coefficient. Then the backward 3-nedian is just a 3/2-nedian in the regular order, and it seems that all his properties are still preserved. Notice that the areas of the 3 and 3/2-nedians are equal, but they are not congruent.
I later found that this idea Professor Satterly calls nedians was written on by Edward John Routh, a famous Mathematical Tripos coach, in 1891 simply using the term cevians. The idea had appeared earlier as a question in the Mathematical Tripos of 1878. Routh's Theorem applies to any ratio between the two parts of a triangle side, even if all three sides are divided in different proportions.
========================================================
Exploring these nedians constructions a little more, I came up with a few more properties that were not in the article. For example, the perpendicular distance from the three vertices of the nedian triangle to any side of ABC will equal the altitude of ABC to that same side. [I call these the sub-altitudes.]
Here the altitude is shown in bold red, and the three corresponding sub-altitudes are shown in dotted red. In the 3-nedian shown the distance from J to side B plus the distance from K to side B plus the distance from L to side B will equal the altitude from B to side B. A similar result exists for each altitude of the triangle. In addition, the three sub-altitudes will always partition the altitude in the same way. The shortest sub-altitude will be%7D%7Bn%5E2-n+1%7Dh "\frac{(1)}{n^2-n+1}h")
, while the next longer one will be %7D%7Bn%5E2-n+1%7Dh "\frac{(n-1)}{n^2-n+1}h")
and the longest will be %5E2%7D%7Bn%5E2-n+1%7Dh "\frac{(n-1)^2}{n^2-n+1}h")
.
It is also clear from the last statement that each of the three small triangles at the vertices of A, B, and C will be congruent. Their bases will each be 1/n of a base of the original triangle and their heights are so each of them is an equal fraction,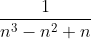 
of the original area of ABC. By similar reasoning we see that all three of the quadrilaterals will also have the same area.
I also observed that that each nedian is partitioned into three parts whose lengths, in order from the vertex to the opposite side, are%5E2-1%7D%7Bn%5E2-n+1%7D,+and+%5Cfrac%7B1%7D%7Bn%5E2-n+1%7D "\frac{n}{n^2-n+1}, \frac{(n-1)^2-1}{n^2-n+1}, and \frac{1}{n^2-n+1}")
.
For the 3-nedians in the image, for example, the nedian CF is partitioned so that CJ is 3/7of CF; JL is 3/7 of CF; and LF is 1/7 of LF. A similar partition holds for the other two 3-nedians AD and BE. In a 4-nedian, the partitions would be 4/14, 8/13, and 1/14.
I wrote recently about the concept of a Nedian; an extension of the idea of medians created by Professor John Satterly of the University of Toronto. I have played around a little more with the concept and come up with a calculation for the area of what I call a nedial triangle, extending the idea of a medial triangle.
Most High School students are introduced to the Medians of a triangle, and it is quite easy to show using basic high school geometry that the triangle whose vertices are the three feet of the medians has an area equal to 1/4 the area of the original triangle. In fact, it is pretty easy to show that the original triangle can be dissected into four congruent copies of the medial triangle.
If instead we use the feet of the three nedians, we get a triangle I have called the nedial triangle. I have worked out that the area of such a triangle for an n-nedian (the cevian that cuts the opposite side 1/n th of the way along the edge) will have an area of
times the area of the original triangle ABC. Since the median is the 2-Nedian, this gives the correct ratio for the medial triangles.
The image below shows the 4-nedial triangle, which has an area of 7/16 the area of ABC.
The area of the three triangles at the vertices of the original triangle will each be
times the area of ABC.
The area of the nedial triangle will grow from a minimum of 1/4 the area of ABC increasing toward a limit of one as n approaches infinity. This can be confirmed by L'Hopital's rule or simple division of the terms. The fact that the limit is also one as n approaches one points out that when the base of the nedian is more than half way along the opposite side and the "n" in the nedian must be less than 2 but more than one we are measuring the area of what Satterly called the "backward nedians" which will create a nedial triangle of the same area. n=3/2 gives the exact area of n=3 (although the triangles are NOT congruent). In general for every k-nedial the area given by the nedial with index equal to n/n-1 has the same area.
Here the altitude is shown in bold red, and the three corresponding sub-altitudes are shown in dotted red. In the 3-nedian shown the distance from J to side B plus the distance from K to side B plus the distance from L to side B will equal the altitude from B to side B. A similar result exists for each altitude of the triangle. In addition, the three sub-altitudes will always partition the altitude in the same way. The shortest sub-altitude will be
It is also clear from the last statement that each of the three small triangles at the vertices of A, B, and C will be congruent. Their bases will each be 1/n of a base of the original triangle and their heights are
I also observed that that each nedian is partitioned into three parts whose lengths, in order from the vertex to the opposite side, are
For the 3-nedians in the image, for example, the nedian CF is partitioned so that CJ is 3/7of CF; JL is 3/7 of CF; and LF is 1/7 of LF. A similar partition holds for the other two 3-nedians AD and BE. In a 4-nedian, the partitions would be 4/14, 8/13, and 1/14.
The Nedial Triangle
I wrote recently about the concept of a Nedian; an extension of the idea of medians created by Professor John Satterly of the University of Toronto. I have played around a little more with the concept and come up with a calculation for the area of what I call a nedial triangle, extending the idea of a medial triangle.
Most High School students are introduced to the Medians of a triangle, and it is quite easy to show using basic high school geometry that the triangle whose vertices are the three feet of the medians has an area equal to 1/4 the area of the original triangle. In fact, it is pretty easy to show that the original triangle can be dissected into four congruent copies of the medial triangle.
If instead we use the feet of the three nedians, we get a triangle I have called the nedial triangle. I have worked out that the area of such a triangle for an n-nedian (the cevian that cuts the opposite side 1/n th of the way along the edge) will have an area of
times the area of the original triangle ABC. Since the median is the 2-Nedian, this gives the correct ratio for the medial triangles.The image below shows the 4-nedial triangle, which has an area of 7/16 the area of ABC.
The area of the three triangles at the vertices of the original triangle will each be
times the area of ABC.The area of the nedial triangle will grow from a minimum of 1/4 the area of ABC increasing toward a limit of one as n approaches infinity. This can be confirmed by L'Hopital's rule or simple division of the terms. The fact that the limit is also one as n approaches one points out that when the base of the nedian is more than half way along the opposite side and the "n" in the nedian must be less than 2 but more than one we are measuring the area of what Satterly called the "backward nedians" which will create a nedial triangle of the same area. n=3/2 gives the exact area of n=3 (although the triangles are NOT congruent). In general for every k-nedial the area given by the nedial with index equal to n/n-1 has the same area.
No comments:
Post a Comment