I had this rare privilege of being able
to pursue in my adult life,
to pursue in my adult life,
what had been my childhood dream.
~Andrew Wiles
The 172nd day of the year; seventeen 2's followed by two 17's is prime.*Prime Curios
222222222222222221717 is prime
172 = pi(1+7+2) * pn{(1*7*2)} . It is the only known number (up to 10^8) with this property.
pi(n) is the number of primes less than or equal to n, and pn is the nth prime.
172/4 = 43, so 44^2 - 42^2 = 172
172/4 = 43, so 44^2 - 42^2 = 172
172 is the sum of Euler's Totient function (the number of smaller numbers for each n, which are coprime to n) over the first 23 integers
172 is the number of pieces a circle can be divided into with 18 straight cuts. It is sometimes called the Lazy Caterer's sequence, and is given by the relation \(p = \frac{n^2+n+2}{2}\)
Since I haven't mentioned this anywhere else yet, these numbers appear in Floyd's Triangle, a programing exercise for beginning programmers which has the Lazy Caterer sequence going veritcally down the altitude of a triangle of numbers, and the triangular numbers on the hypotenuse
1
2, 3
4, 5, 6
7, 8, 9, 10
11.....
 |
| *Wik |
172 is a repdigit in base 6(444), and also in base 42 (44)
1667 Louis XIV, The Sun King of France, attends the ceremony of inauguration of the Observatoire de Paris, the oldest working observatory in the world. *Amir D. Aczel, Pendulum, pg 66
The Paris Observatory (French: Observatoire de Paris; a research institution of the Paris Sciences et Lettres University, is the foremost astronomical observatory of France, and one of the largest astronomical centers in the world. Its historic building is on the Left Bank of the Seine in central Paris, but most of the staff work on a satellite campus in Meudon, a suburb southwest of Paris.
The Paris Observatory at the beginning of the eighteenth century, with the wooden "Marly Tower" on the right, a remnant of the Machine de Marly moved to the grounds by Giovanni Cassini, for the mounting of long-tubed telescopes and even longer tubeless aerial telescopes.
1669 Christopher Wren gives first proof that the hyperboloid of one sheet (Wren uses the term Hyperbolic Cylindroid.) is doubly ruled in the Philosophical Transactions of the Royal Society. The only three doubly ruled surfaces are the plane, the hyperboloid of one sheet, and the hyperbolic paraboloid. Wren includes an image of the hyperboloid of one sheet that may be the earliest ever in print. In a footnote in Boyer's History of History of Analytic Geometry he notes that there is a figure in Kepler's Stereometria which looks like it might be this shape. (It is interesting that in his work on the geometry of a barrel, Kepler gives an approximation formula for the volume of a barrel that is exact for the hyperboloid of one sheet.)
The invention of the telescope and efforts to reduce distortion in the lenses led to suggestions of hyperbolic lenses, and Wren's paper pointed out "an application thereof for grinding hyperbolical glasses." Newton had applied the knowledge that the hyperboloid of one sheet was doubly ruled in his notes in 1666 when he demonstrated how to turn the shape on a lathe holding the cutting tool obliquely to the axis of rotation.
The image of Newton's method below is from a paper by Professor Rickey on the net.
*Wik, *VFR,
1798 Cavendish reads a paper to the Royal Society of London describing experiments to measure the density of the earth, and hence its weight, with results that it is 5.48 times the density of water. (the figures seem to include at least one calculating error) *Philosophical Transactions, 1798, Part II, pgs 469-526
1808 on 30 June, Humphry Davy announced he had separated the element boron. However, working independently, French chemist, Joseph Louis Gay-Lussac had announced* the same accomplishment nine days earlier, on 21 Jun 1808*TIS
1838 The earliest stereoscopes, "both with reflecting mirrors and with refracting prisms", were invented by Sir Charles Wheatstone and constructed for him by optician R. Murray in 1832. Herbert Mayo shortly described Wheatstone's discovery in his book Outlines of Human Physiology (1833) and claimed that Wheatstone was about to publish an essay about it. It was only one of many projects of Wheatstone's and he first presented his findings on 21 June 1838 to the Royal College of London.
In this presentation he used a pair of mirrors at 45 degree angles to the user's eyes, each reflecting a picture located off to the side. It demonstrated the importance of binocular depth perception by showing that when two pictures simulating left-eye and right-eye views of the same object are presented so that each eye sees only the image designed for it, but apparently in the same location, the brain will fuse the two and accept them as a view of one solid three-dimensional object. Wheatstone's stereoscope was introduced in the year before the first practical photographic processes became available, so initially drawings were used. The mirror type of stereoscope has the advantage that the two pictures can be very large if desired.
In 1886, the foundation stone of the Tower Bridge in London, England was laid (over a time capsule) by the Prince of Wales. The need to cross the River Thames at this point had become increasingly urgent for many years, and finally the necessary Act was passed in 1885. The bridge, designed by Mr. Wolfe Barry, CB, was completed at a cost of about £1,000,000. To permit the passage of tall ships between the towers, two bascule spans, each of 100-ft length, are raised. The side spans to the towers are of the more familiar suspension type. Pedestrians can traverse a high-level footway nearly at the top of the towers, even when the bridge is raised. It was officially opened 30 Jun 1894, by the Prince of Wales, later Edward VII, on behalf of Queen *TIS
 |
| *wik |
In 1893, the first Ferris wheel premiered at Chicago's Colombian Exposition, America's third world's fair. It was invented by George Washington Ferris, a Pittsburgh bridge builder, for the purpose of creating an attraction like the Eiffel Tower in Paris. Each of the 36 cars carried 60 passengers, making a full passenger load of 150 tons. Ferris didn't use rigid spokes: instead, he used a web of taut cables, like a bicycle wheel. Supported by two 140 foot steel towers, its 45 foot axle was the largest single piece of forged steel at the time in the world. The highest point of the wheel was 264 feet. The wheel and cars weighed 2100 tons, with another 2200 tons of associated levers and machinery. Ferris died just four years later, at the age of only 38. *TIS
"Pleasure wheels", whose passengers rode in chairs suspended from large wooden rings turned by strong men, may have originated in 17th-century Bulgaria. *Wik
 |
| The Original Ferris Wheel *Wik |
1929 Kazimierz Kuratowski (1896–1980) at a meeting of the Warsaw Section of the Polish Mathematical Society, announced that a graph is planar iff it does not contain a subgraph homeomorphic to either K–5, the complete graph on 5 points, or K–3–3, the complete bipartite graph on two sets of three points. See HM 12, 258, for a discussion of the early history of this theorem which is now the most cited result in graph theory. *VFR (See June 18)
"A finite graph is planar if and only if it does not contain a subgraph that is a subdivision of K5 (the complete graph on five vertices) or K3,3 (complete bipartite graph on six vertices, three of which connect to each of the other three)." *Wik
(in more simple, but less exact terms, "it can be drawn in such a way that no edges cross each other." The well-known recreational problem of connecting three houses to three utilities is not possible to draw because it is K3,3 (below). The utility problem posits three houses and three utility companies--say, gas, electric, and water--and asks if each utility can be connected to each house without having any of the gas/water/electric lines/pipes pass over any other. (1913 Dudeney: first publication of Gas, Water and Electricity Problem. according to David Singmaster, Gardner says 1917)
(in more simple, but less exact terms, "it can be drawn in such a way that no edges cross each other." The well-known recreational problem of connecting three houses to three utilities is not possible to draw because it is K3,3 (below). The utility problem posits three houses and three utility companies--say, gas, electric, and water--and asks if each utility can be connected to each house without having any of the gas/water/electric lines/pipes pass over any other. (1913 Dudeney: first publication of Gas, Water and Electricity Problem. according to David Singmaster, Gardner says 1917)
(1913 Dudeney: first publication of Gas, Water and Electricity Problem. according to David Singmaster, Gardner says 1917) [Since Kuratowski was 15 years old at this time, it could not have been a proof of the houses and utilities problem then, however it could have been proven by the Gem of Euler, (V - E + F = 2). My version is here. *PB ]
1948 the first stored-program computer, the Small-Scale Experimental Machine, SSEM, ran its first program. Written by Professor Tom Kilburn, it took 52 minutes to run. The tiny experimental computer had no keyboard or printer, but it successfully tested a memory system developed at Manchester University in England. The system, based on a cathode-ray tube, could store programs. Previous electronic computers had to be rewired to execute each new problem. The Manchester computer proved theories set forth by John von Neumann in a report that proposed modifications to ENIAC, the electronic computer built at the University of Pennsylvania in the mid-1940s. The report also proposed the use of binary instead of digital numbers. *TIS
1963 A brief note about the introduction of the Friden 6010 Computyper business computer system in the June, 21, 1963 edition of Electronics magazine. The 6010 was a small-scale desk-sized computing system with plug-board and tab-rack controlled programming/sequencing, as well as magnetic core memory for storage registers, and an electronic math unit for performing fixed point addition, subtraction, multiplication and division. The primary input to the machine was eight-channel punched paper tape or ledger cards, with human input through the keyboard of the included Friden Flexowriter. Output could be typewritten via the Flexowriter, or to punched tape or ledger cards via the Flexowriter's eight-channel tape punch. Later, various peripheral devices were added to the system's options including magnetic tape, and even a removable platter disk drive system.
It is one of the earliest all-electronic desktop calculators, and is generally regarded as the first solid-state transistorized electronic calculator, although there is evidence that Sharp (Compet 10) and IME (IME 84) actually introduced their first electronic calculator just days before Friden did.
1976 Kenneth Appel and Wolfgang Haken announced that with the aid of a computer that they had proved the four color problem. Because of the use of the computer the solution was not quickly accepted by all, but today most mathematicians accept the proof as correct. However, no simple proof is known as yet. *VFR
In 1963 Donald B. Gillies had found three new primes. When the primes were confirmed the UIUC Math dept (which has a postal branch) used this cancellation stamp on all mail from roughly 1964 - 1976. When Appel and Haken proved the four color theorem ("Four Colors Suffice") a new stamp was created. Trivia question : how far away from Gillies did Appel live in Urbana Illinois ??
Answer : He lived 3 houses away. *Wik
Answer : He lived 3 houses away. *Wik
 |
| *Wik courtesy of Chris Caldwell |
1993 Andrew Wiles begins the three days of lectures leading to a solution of Taniyama-Shimura conjecture, and completing the proof of Fermat’s last theorem.. See (June 23)
2023 On non-leap years (until 2039), this day marks the summer solstice in the northern hemisphere and the winter solstice in the southern hemisphere, and this is the day of the year with the longest hours of daylight in the northern hemisphere and the shortest in the southern hemisphere. On Leap years it happens a day earlier.*Wik
2023 On non-leap years (until 2039), this day marks the summer solstice in the northern hemisphere and the winter solstice in the southern hemisphere, and this is the day of the year with the longest hours of daylight in the northern hemisphere and the shortest in the southern hemisphere. On Leap years it happens a day earlier.*Wik
BIRTHS
1710 James Short (June 10 {June 21 NS), 1710, Edinburgh, Scot. - June 14, 1768, London, Eng) British optician and astronomer who produced the first truly
1781 Siméon-Denis Poisson ( 21 June 1781 – 25 April 1840) French mathematician known for his work on definite integrals, advances in Fourier series, electromagnetic theory, and probability. The Poisson distribution (1837) describes the probability that a random event will occur in a time or space interval under the conditions that the probability of the event occurring is very small, but the number of trials is very large so that the event actually occurs a few times. His works included applications to electricity and magnetism, and astronomy. He is also known for the Poisson's integral, Poisson's equation in potential theory, Poisson brackets in differential equations, Poisson's ratio in elasticity, and Poisson's constant in electricity.*TIS Libri wrote of him: “His only passion has been science: he lived and is dead for it.” *VFR
1857 Hugh Frank Newall, FRS FRAS (21 June 1857 – 22 February 1944) was a British astrophysicist. Newall held the first chair of astrophysics at Cambridge University (1909-1928). After teaching at Wellington College, he went to Cambridge to be an assistant to J. J. Thomson. He changed his interests from being senior demonstrator in experimental physics to astronomy when he facilitated the university's acquisition of the 25-inch Newall Telescope after the death of his father, Robert Stirling Newall, in 1889. His father, an engineer in manufacturing wire ropes and submarine telegraph cables, had the telescope built for private use at his Gateshead home. Hugh paid the moving expenses. When built, it was the largest in the world, and remained so for many years. He designed spectrographs and studied the solar corona, became director of the Solar Physics Observatory (1913) and led many eclipse expeditions. *TiS
1863 Maximilian Franz Joseph Cornelius Wolf ( 21 June 1863 – 3 October 1932) was a German astronomer who founded and directed the Königstuhl Observatory. He used wide-field photography to study the Milky Way and used statistical treatment of star counts to prove the existence of clouds of dark matter. He was among the first astronomers to show that the spiral nebulae have absorption spectra typical of stars and thus differ from gaseous nebulae. His most important contribution was the introduction of photography to discover hundreds of asteroids, the first of which he named Brucia in honor of the donor of his 16-inch double telescope, Catherine Wolfe Bruce.*TIS
1870 Clara Helene Immerwahr (21 June 1870 – 2 May 1915) was a German chemist. She was the first German woman to be awarded a doctorate in chemistry from the University of Breslau, and is credited with being a pacifist as well as a "heroine of the women's rights movement". From 1901 until her suicide in 1915, she was married to the Nobel Prize-winning chemist Fritz Haber.
Due to societal expectations that a married woman's place was in the home, her ability to conduct research was limited. She instead contributed to her husband's work with minimal recognition, translating some of his papers into English. On 1 June 1902 she gave birth to Hermann Haber (1902–1946), the only child of that marriage.
Confiding in Abegg, Immerwahr expressed her deep dissatisfaction with this subservient role:
It has always been my attitude that a life has only been worth living if one has made full use of all one's abilities and tried to live out every kind of experience human life has to offer. It was under that impulse, among other things, that I decided to get married at that time... The life I got from it was very brief...and the main reasons for that was Fritz's oppressive way of putting himself first in our home and marriage, so that a less ruthlessly self-assertive personality was simply destroyed.
 |
| *Wik |
1876 Willem Hendrik Keesom (21 June 1876, Texel – 3 March 1956, Leiden) Dutch physicist who was a pioneer in cryogenics and was the first to solidify helium under pressure (1926). He was a research assistant for Kamerlingh Onnes working on the liquefaction of helium, and several years later, subsequently succeeded him (1923) as director of the Physics Laboratory at Leiden. In work done with M. Wolfke, after studying discontinuities in several properties of helium at very low temperatures (1927) they suggested that it may be due to a phase change. They called the helium above the transitional helium I and the helium below the transition helium II. In 1932, he produced a temperature just two degrees above absolute zero (-272° C or -457.6° F). In 1942 he wrote the book Helium.*TiS
1916 Herbert Friedman (June 21, 1916 – September 9, 2000) American astronomer who made seminal contributions to the study of solar radiation. He joined the Naval Research Laboratory in 1940 and developed defense-related radiation detection devices during WW II. In 1949, he obtained the first scientific proof that X rays emanate from the sun. When he directed the firing into space of a V-2 rocket carrying a detecting instrument. Through rocket astronomy, he also produced the first ultraviolet map of celestial bodies, and gathered information for the theory that stars are being continuously formed, on space radiation affecting Earth and on the nature of gases in space. He also made fundamental advances in the application of x rays to material analysis.*TiS
1918 Tibor Szele (21 June 1918 – 5 April 1955) worked in group theory. *VFR Hungarian mathematician, working in combinatorics and abstract algebra. After graduating at the Debrecen University, he became a researcher at the Szeged University in 1946, then he went back to Debrecen University in 1948 where he became full professor in 1952. He worked especially in the theory of Abelian groups and ring theory. He generalized Hajós's theorem. He founded the Hungarian school of algebra. *Wik
1954 David Ríos Insua (born June 21, 1964 in Madrid) is a Spanish mathematician, and son and disciple of Sixto Ríos, father of Spanish Statistics. He is currently also the youngest Fellow of the Spanish Royal Academy of Sciences (de la Real Academia de Ciencias Exactas, Físicas y Naturales, RAC), which he joined in 2008. He received a PhD in Computational Sciences at the University of Leeds. He is Full Professor of the Statistics and Operations Research Department at Rey Juan Carlos University (URJC), and he has been Vice-dean of New Technologies and International Relationships at URJC (2002–2009). He has worked in fields such as Bayesian inference in neuronal networks, MCMC methods in decision analysis, Bayesian robustness or adversarial risk analysis. He has also worked in applied areas such as Electronic Democracy, reservoirs management, counterterrorism model and many others. He is married and has two daughters. Wik
DEATHS
1820 Alexis Thérèse Petit (2 October 1791, Vesoul, Haute-Saône – 21 June 1820, Paris) was a French physicist.
Petit is known for his work on the efficiencies of air- and steam-engines, published in 1818 (Mémoire sur l’emploi du principe des forces vives dans le calcul des machines). His well-known discussions with the French physicist Sadi Carnot, founder of thermodynamics, may have stimulated Carnot in his reflexions on heat engines and thermodynamic efficiency. The Dulong–Petit law (1819) is named after him and his collaborator Pierre Louis Dulong.
1913 Gaston Tarry (27 September 1843 – 21 June 1913) was a French combinatorialist whose best-known work is a method for solving mazes.*SAU He also was able to confirm Leonhard Euler's conjecture that no 6×6 Graeco-Latin square was possible.
In mathematics, the Prouhet–Tarry–Escott problem asks for two disjoint sets A and B of n integers each, such that:
For example, a solution with n = 6 and k = 5 is the two sets { 0, 5, 6, 16, 17, 22 } and { 1, 2, 10, 12, 20, 21 }, because:
- 01 + 51 + 61 + 161 + 171 + 221 = 11 + 21 + 101 + 121 + 201 + 211
- 02 + 52 + 62 + 162 + 172 + 222 = 12 + 22 + 102 + 122 + 202 + 212
- 03 + 53 + 63 + 163 + 173 + 223 = 13 + 23 + 103 + 123 + 203 + 213
- 04 + 54 + 64 + 164 + 174 + 224 = 14 + 24 + 104 + 124 + 204 + 214
- 05 + 55 + 65 + 165 + 175 + 225 = 15 + 25 + 105 + 125 + 205 + 215.
1940 Wolfgang Döblin, known in France as Vincent Doblin, (17 March 1915 – 21 June 1940) was a German-French mathematician. Wolfgang was the son of the Jewish-German novelist, Alfred Döblin. His family escaped from Nazi Germany to France where he became a citizen. Studying probability theory at the Institute Henri Poincaré under Fréchet, he quickly made a name for himself as a gifted theorist. He became a doctor at age 23. Drafted in November 1938, after refusing to be exempted of military service, he had to stay in the active Army when World War II broke out in 1939, and was quartered at Givet, in the Ardennes, as a telephone operator. There, he wrote down his latest work on the Chapman-Kolmogorov equation, and sent this as a "pli cacheté" (sealed envelope) to the French Academy of Sciences. His company, sent to the sector of the Saare on the ligne Maginot in April 1940, was caught in the German attack in the Ardennes in May, withdrew to the Vosges, and capitulated on June 22, 1940. On June 21, Doeblin had committed suicide in Housseras (a small village near to Epinal), at the moment where German troops came in sight of the place. In his last moments, he burned his mathematical notes.
The sealed envelope was opened in 2000, revealing that Döblin was ahead of his time in the development of the theory of Markov processes. In recognition of his results, Itō's lemma is now referred to as the Itō–Doeblin Theorem.
His life was recently the subject of a movie by Agnes Handwerk and Harrie Willems, A Mathematician Rediscovered. *Wik
1948 Sir D'Arcy Wentworth Thompson CB FRS FRSE (2 May 1860 – 21 June 1948) graduated from Cambridge University in Zoology. He was a appointed Professor of Biology at Dundee and later Professor of Natural History at St Andrews. He combined skills in a way that made him unique. He was a Greek scholar, a naturalist and a mathematician. He was the first biomathematician. He became an honorary member of the EMS in 1933. *SAU
1957 Johannes Stark ( 15 April 1874 – 21 June 1957) was a German physicist who was awarded the Nobel Prize in Physics in 1919 "for his discovery of the Doppler effect in canal rays and the splitting of spectral lines in electric fields". This phenomenon is known as the Stark effect.
Stark received his Ph.D. in physics from the University of Munich in 1897 under the supervision of Eugen von Lommel, and served as Lommel's assistant until his appointment as a lecturer at the University of Göttingen in 1900. He was an extraordinary professor at Leibniz University Hannover from 1906 until he became a professor at RWTH Aachen University in 1909. In 1917, he became professor at the University of Greifswald, and he also worked at the University of Würzburg from 1920 to 1922.
A supporter of Adolf Hitler from 1924, Stark was one of the main figures, along with fellow Nobel laureate Philipp Lenard, in the anti-Semitic Deutsche Physik movement, which sought to remove Jewish scientists from German physics. He was appointed head of the German Research Foundation in 1933 and was president of the Reich Physical-Technical Institute from 1933 to 1939. In 1947 he was found guilty as a "Major Offender" by a denazification court. *Wik
Credits :
*CHM=Computer History Museum
*FFF=Kane, Famous First Facts
*NSEC= NASA Solar Eclipse Calendar
*RMAT= The Renaissance Mathematicus, Thony Christie
*SAU=St Andrews Univ. Math History
*TIA = Today in Astronomy
*TIS= Today in Science History
*VFR = V Frederick Rickey, USMA
*Wik = Wikipedia
*WM = Women of Mathematics, Grinstein & Campbell
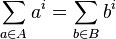
No comments:
Post a Comment